Overview
The futures market offers trading instruments on a very diverse range of underlying assets, including precious metals, agricultural commodities, oil and gas, equity, interest rates, volatility, cryptocurrencies, and more. As a consequence, professional futures portfolio managers, commonly known as Commodity Trading Advisors (CTAs), now have access to various assets not only within the same asset class (e.g. gold, silver, and copper), but also across asset classes (e.g. precious metals and volatility).
Futures portfolios with multiple underlying assets give rise to some new challenges. With more assets and trading instruments, the dimension of the portfolio optimization problem is significantly increased. Furthermore, one must address the dependency structure among the underlying assets and their futures, even if the underlying assets are not traded.
This calls for a stochastic model that can capture the correlation among all the futures and underlying assets while maintaining analytical tractability, numerical efficiency, and interpretability. Motivated by these observations, we introduce in this paper an alternative way to model the joint price dynamics of the underlying assets and associated futures.
Methodology
The key idea is as follows. In practice, market frictions and inefficiencies may render the futures price different from the spot price prior to maturity. For each futures contract, the spread between the two prices is called the basis. By no-arbitrage theory, futures prices must converge to the spot price at expiry, so the basis process is expected to converge to zero as the associated futures contract expires as well. This price behavior leads us to (i) express each futures price process through the associated basis, and (ii) model the random basis using a Brownian bridge. For multiple futures contracts, we consider a multidimensional Brownian bridge, where each component converges to zero at the respective maturity.
In our new article, Constrained Dynamic Futures Portfolios with Stochastic Basis (see also References below), we analyze the problem of dynamically trading futures with different underlying assets under the stochastic basis model driven by a multidimensional Brownian bridge.
Another key element of our utility maximization problem is the incorporation of portfolio constraints on the futures positions. Our general portfolio setup captures the dollar neutral and market neutral constraints, which are widely used in industry. The optimal strategies for both unconstrained and constrained cases are derived. This is achieved by solving the associated Hamilton-Jacobi-Bellman (HJB) equations. Moreover, we show that the original nonlinear HJB equations are reduced to a system of linear ODEs that can be solved instantly, which in turn generate the optimal futures positions.
Numerical Example
In the paper, we present a numerical example to illustrate the results. We consider a market with two different assets and three futures. The trading horizon is set to be strictly less than the futures maturities.
In Figure 1, we display the simulated paths of the underlying asset price, futures price, and corresponding log-bases. In Figure 2, we show the terminal wealth distribution, based on Monte Carlo simulation of the stochastic basis model. In this example, we observe that the portfolio constraint can effectively reduce the tails (and standard deviation) of the wealth distribution.
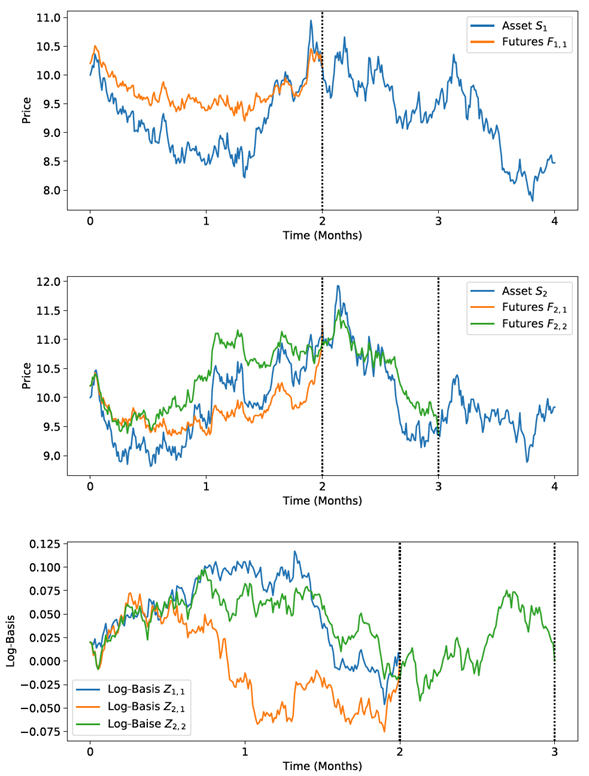
Fig. 1: Simulated paths of the underlying asset price, futures price, and corresponding log-bases. Source: Chen et. al (2021) (see References below)
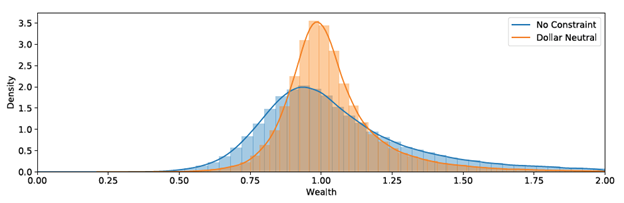
Fig. 2: The distributions of terminal wealth (based on Monte Carlo simulation) for the unconstrained and constrained portfolios. Source: Chen et. al (2021) (see References below)
References:
Xiaodong Chen, Tim Leung, and Yang Zhou (2021), Constrained Dynamic Futures Portfolios with Stochastic Basis [pdf], to appear, Annals of Finance
Related Articles:
T. Leung and B. Angoshtari (2021), Optimal Trading of a Basket of Futures Contracts [pdf], Vol.16, pages 253–280, Annals of Finance
T. Leung and Y. Zhou (2021), Optimal Dynamic Futures Portfolio Under a Multifactor Gaussian Framework [pdf], Vol. 24, No. 5, p.2150028, International Journal of Theoretical & Applied Finance
Disclosure: Interactive Brokers
Information posted on IBKR Campus that is provided by third-parties does NOT constitute a recommendation that you should contract for the services of that third party. Third-party participants who contribute to IBKR Campus are independent of Interactive Brokers and Interactive Brokers does not make any representations or warranties concerning the services offered, their past or future performance, or the accuracy of the information provided by the third party. Past performance is no guarantee of future results.
This material is from Computational Finance & Risk Management, University of Washington and is being posted with its permission. The views expressed in this material are solely those of the author and/or Computational Finance & Risk Management, University of Washington and Interactive Brokers is not endorsing or recommending any investment or trading discussed in the material. This material is not and should not be construed as an offer to buy or sell any security. It should not be construed as research or investment advice or a recommendation to buy, sell or hold any security or commodity. This material does not and is not intended to take into account the particular financial conditions, investment objectives or requirements of individual customers. Before acting on this material, you should consider whether it is suitable for your particular circumstances and, as necessary, seek professional advice.
Disclosure: Bitcoin Futures
TRADING IN BITCOIN FUTURES IS ESPECIALLY RISKY AND IS ONLY FOR CLIENTS WITH A HIGH RISK TOLERANCE AND THE FINANCIAL ABILITY TO SUSTAIN LOSSES. More information about the risk of trading Bitcoin products can be found on the IBKR website. If you're new to bitcoin, or futures in general, see Introduction to Bitcoin Futures.
Disclosure: Futures Trading
Futures are not suitable for all investors. The amount you may lose may be greater than your initial investment. Before trading futures, please read the CFTC Risk Disclosure. A copy and additional information are available at ibkr.com.